
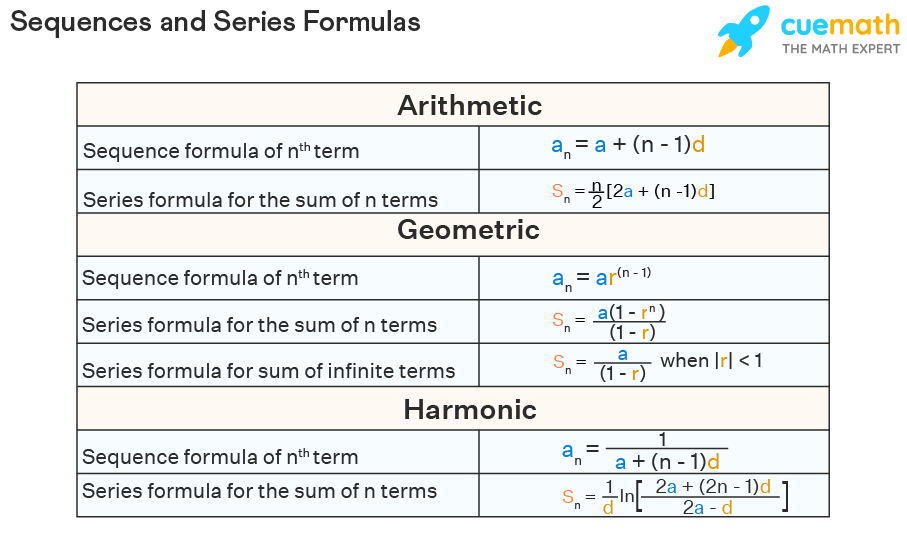
is the sum of the terms of an arithmetic sequence. In some cases, the first term of an arithmetic sequence may not be given.Īn arithmetic series The sum of the terms of an arithmetic sequence.
Next, use the first term a 1 = − 8 and the common difference d = 3 to find an equation for the nth term of the sequence.Ī n = − 8 + ( n − 1 ) ⋅ 3 = − 8 + 3 n − 3 = − 11 + 3 n Substitute a 1 = − 8 and a 7 = 10 into the above equation and then solve for the common difference d. In this case, we are given the first and seventh term:Ī n = a 1 + ( n − 1 ) d U s e n = 7. In other words, find all arithmetic means between the 1 st and 7 th terms.īegin by finding the common difference d. In fact, any general term that is linear in n defines an arithmetic sequence.įind all terms in between a 1 = − 8 and a 7 = 10 of an arithmetic sequence. In general, given the first term a 1 of an arithmetic sequence and its common difference d, we can write the following:Ī 2 = a 1 + d a 3 = a 2 + d = ( a 1 + d ) + d = a 1 + 2 d a 4 = a 3 + d = ( a 1 + 2 d ) + d = a 1 + 3 d a 5 = a 4 + d = ( a 1 + 3 d ) + d = a 1 + 4 d ⋮įrom this we see that any arithmetic sequence can be written in terms of its first element, common difference, and index as follows:Ī n = a 1 + ( n − 1 ) d A r i t h m e t i c S e q u e n c e Here a 1 = 1 and the difference between any two successive terms is 2. For example, the sequence of positive odd integers is an arithmetic sequence, Find the roots of the polynomial, given that the roots form an arithmetic progression.An arithmetic sequence A sequence of numbers where each successive number is the sum of the previous number and some constant d., or arithmetic progression Used when referring to an arithmetic sequence., is a sequence of numbers where each successive number is the sum of the previous number and some constant d.Ī n = a n − 1 + d A r i t h m e t i c S e q u e n c eĪnd because a n − a n − 1 = d, the constant d is called the common difference The constant d that is obtained from subtracting any two successive terms of an arithmetic sequence a n − a n − 1 = d.Here are some problems with solutions that utilize arithmetic sequences and series. Then by the above formula, the series has value This completes the proof. The second is that if an arithmetic series has first term, common difference, and terms, it has value. Then, we can write in two ways: Adding these two equations cancels all terms involving and so, as required. Proof: Let the series be equal to, and let its common difference be. The first is that if an arithmetic series has first term, last term, and total terms, then its value is equal to. As for finite series, there are two primary formulas used to compute their value. This is mostly used to perform substitutions, though it occasionally serves as a definition of arithmetic sequences.Īn arithmetic series is the sum of all the terms of an arithmetic sequence.
Then using the above result, as desired.Īnother common lemma is that a sequence is in arithmetic progression if and only if is the arithmetic mean of and for any consecutive terms. Proof: Let the sequence have first term and common difference. Ī common lemma is that given the th term and th term of an arithmetic sequence, the common difference is equal to. Let be the first term, be the th term, and be the common difference of any arithmetic sequence then. Because each term is a common distance from the one before it, every term of an arithmetic sequence can be expressed as the sum of the first term and a multiple of the common difference.


 0 kommentar(er)
0 kommentar(er)
